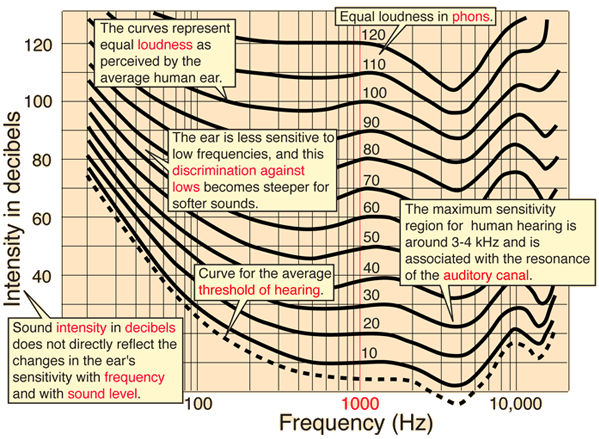
Equal Loudness Curves

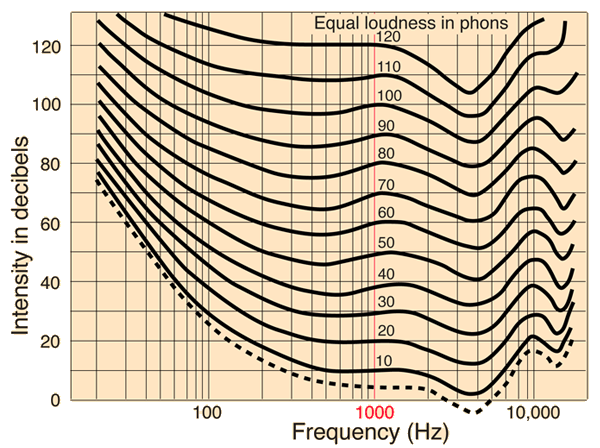
Fletcher and Munson are credited with pioneering work in the 1930s to develop equal-loudness curves, contributing significantly to the understanding of the loudness response of the human ear. Sets of equal-loudness contours are still often referred to as Fletcher-Munson curves. The above curves follow the equal-loudness curves resulting from the measurements of Robinson and Dadson in the mid 1950s and are adapted from the depiction of the curves in Donald Hall's book "Musical Acoustics". Subsequent measurements of the human ear's loudness response were standardized under the designation ISO 226 Standard.

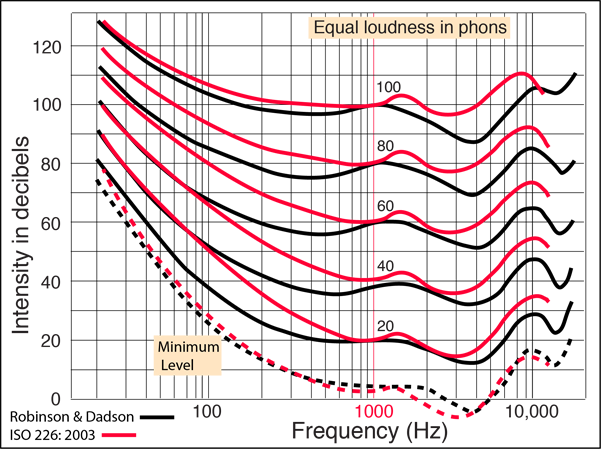
This illustration seeks to show the difference between the more recent ISO 226:2003 set of equal-loudness curves and the Robinson & Dadson curves. Most notable is the fact that the curves are steeper in the low to mid loudness levels for low frequencies. The measurements were made with individuals of normal hearing in the 18-25 year age range.

Annotated Equal Loudness Curves

Example of Information from Equal Loudness Curves

Three example curves from the equal loudness curves are shown below, corresponding to very soft, midrange and very loud sounds.

Examination of these three curves makes it evident that there is considerable difference between the ear's response at different sound levels. The response to very loud sounds is much "flatter" or more uniform than the response to very soft sounds, although it still shows the prominent enhancement of sensitivity between about 2000-5000Hz associated with the ear canal resonance. Where the curve dips between 2000-5000Hz, this implies that less sound intensity is necessary for the ear to perceive the same loudness as a 120dB, 1000Hz tone. In contrast, the strong rise in the curve for 0 phons at low frequencies shows that the ear has a notable discrimination against low frequencies for very soft sounds.

Since the vertical axis is in decibels, the flat horizontal line at 65dB represents an equal intensity at all frequencies. The example sounds A, B, C and D all have the same sound intensity of 65dB. However, this does not imply that they have the same loudness to the human ear. We can say that sounds A and D have the same loudness since both are on the same equal loudness curve. This curve passes through 60dB at 1000Hz, so we characterize all sounds on that equal loudness curve, including sounds A and D, as having a loudness of 60 phons. Sound B is above the 60 phon curve, so that implies that it would be perceived as louder than A or D. In fact, since sound B is at 1000Hz and has an intensity of 65 dB, we can say that its loudness is 65 phons. The perceived loudness at 1000 Hz is the reference point for defining the equal loudness curve through that point, so the numerical value of phons and dB is always the same at 1000 Hz. Finally, we could say that sound C at 65dB is the loudest of the four sounds since it shows the greatest displacement above the 60 phon curve. From this graph, we cannot determine what that phon level is; that would require experimental comparison with 1000 Hz tones.