Observer Coordinate Systems

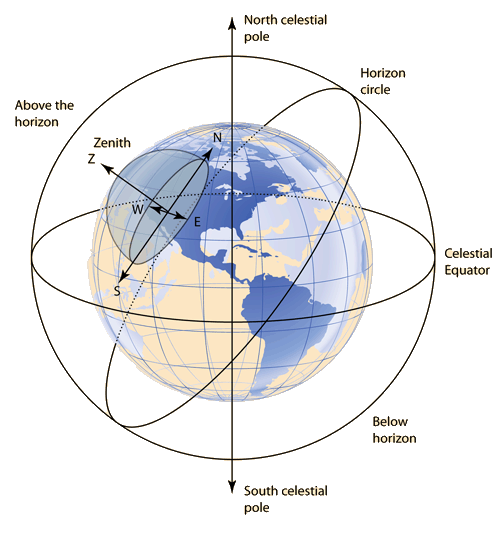
From an observer's position on the Earth, one can define a coordinate system based on the horizon circle and the compass directions.

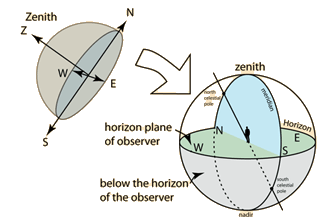
From a horizon plane coordinate system anywhere on the Earth's, we can construct an ordinary observer's reference frame with a zenith overhead and compass directions in the horizontal plane. From the surface observer's point of view, the zenith and Polaris (defining the celestial north pole) are convenient reference points.

The plane through the north celestial pole and the zenith is called the celestial meridian or commonly just the meridian, understood to be this specific meridian. When an object crosses this meridian, it is called its meridian transit and it will be highest in the sky at that point.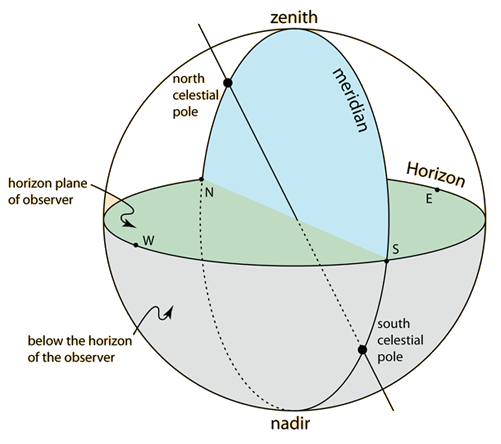

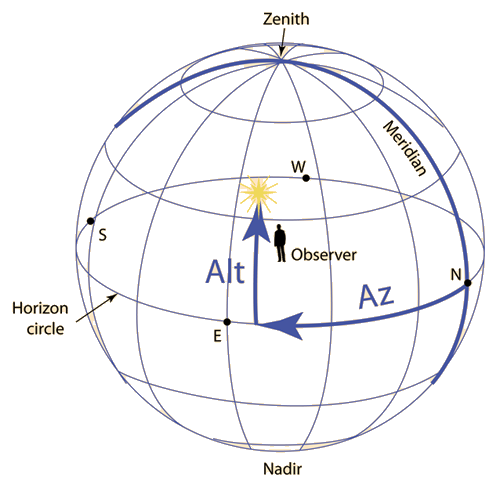
For pointing a telescope, it is convenient to use the Azimuth angle Az from North toward East and the Altitude angle Alt from the horizon plane. Also used is the zenith angle z, which is just 90° - Alt.