Nuclear Radiation and Nuclear Decay
I. The Decay of Radioactive Waste Products
You have in your possession a 1000 liter tank of radioactively contaminated water. Before you can discharge this water, the radioactive content must be down to 1 x 10-6 curies/liter (1 microcurie per liter). The object of this exercise is to calculate the time you must hold the material before discharge. A calculation routine in HyperPhysics will help you with the calculation.
You will be given data on the amounts and halflives of the radioactive contaminants. If the amount of a radioactive substance in curies at a starting time is A0, then the amount at any time t after that is given by the relationship
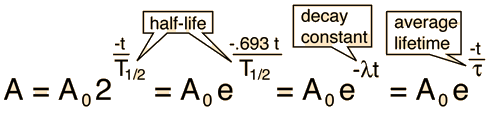
where T is the halflife of the material. Since different contaminants decay with different halflives, this relationship must be applied independently to the different constituents.
Your report for this segment will consist of the lists of your radioisotopes, their halflives and original amounts, the time required for the total contamination to drop below 0.001 curie, and the amounts of the various constituents lift at that time. Once you have obtained the halflives of each of your contaminants, calculate the time required for each to drop to the required limit individually. The longest time that you get is your estimate of the holding time required.
The radioisotopes and their halflives used in this experiment are actual data for these substances. The only thing which is not realistic about the calculations done is that it is assumed that after the radioactive decay the material is stable. In fact, many of the daughter products are themselves radioactive and their activity must be taken into account in actual radioactive waste disposal calculations.
II. Radioactive Dating
Since radioactive isotopes follow a well established law as they decay in time, their decay processes can be used as long-term clocks to measure the time involved in geologic processes which may span millions of years. In the case of carbon-14, with a halflife of about 5730 years, the carbon-dating process can be used to measure shorter-term processes over periods up to 20,000 years.
If only two constituents A and B are involved such that A decays to form B, and all the material was substance A when the process started, the decay process proceeds as illustrated:
With these assumptions, if you dug up a sample which was three-fourths B and one-fourth A, you could conclude that it had been there two halflives. If A were potassium-40 and B were Argon, then you would conclude that it had been there 2.6 billion years (the halflife of potassium-40 is 1.3 billion years).
The usual case would be that you could not assume that there was none of substance B present at the time of deposition of the material. The practical dating cases involve two or more radioactive processes in the same sample so that assumptions about original composition could be varied until all radioactive processes in the sample converge to the the same age.
In this exercise you will be given some data and asked to find the age. First, find it within one halflife as in the following example:
A: 10 gm, B: 60 gm, halflife 10 years
Estimate: A is 1/7 of the total, which is between 1/4 and 1/8, so the age is more than two but less than three halflives, or between 20 and 30 years.
After you have made your estimate, find the age more accurately using the halflife calculation. This computer routine uses the relationship
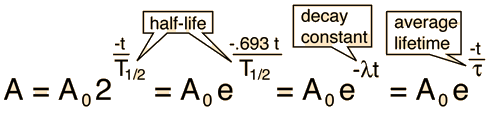
For the above example this relationship gives t = 28.07 years.
Your report for this segment of the experiment consists of the original data given you, your approximate time calculation, and the computer calculated time.
III. Simulated Brain Scan
Radioactive isotopes are used in a variety of diagnostic scanning procedures. The idea is to find a radioactive "tracer" which will be selectively taken up by an organ or area of interest while surrounding tissue stays relatively free of the tracer. The radioactive emissions from the tracer, usually gamma rays, then penetrate the body and are detected by a sensitive radiation detector outside the body to form an image of the organ or object of interest. These scans include thyroid, lung, liver, bone, and brain scans in an ever-growing list. We have chosen to simulate a brain scan since it probably is the best known application of nuclear radiation to diagnostic medicine.
A high degree of accuracy and patient safety combined with technical simplicity has made brain-scanning an accepted diagnostic tool in patients suspected of mass lesions of the brain. The physiological mechanism which makes brain scanning feasible is the so-called "blood-brain barrier". The normal brain tissue is relatively impermeable to most substances: thus there is a low uptake of radioactive tracer by the normal brain tissue. Tracers readily diffuse into the blood pathways of lesions such as tumors, which can then be detected against the low activity of the normal brain tissue.
The "patient" in this experiment is a silhouette of a head. Your patient has a tumor that has absorbed a radioactive tracer element. The actual "tumor" is a piece of a cloth mantle from a camping lantern -- these mantles have a small amount of radioactive thorium which causes the mantle to glow for chemical reasons unrelated to the actual radioactivity.
Halflives of Selected Radioisotopes
- Polonium-218 3.05 minutes
- Radium 1622 years
- Sodium-22 2.58 years
- Cesium-137 30 years
- Strontium-85 64 days
- Strontium-90 28 years
- Iodine-131 8 days
- Phosphorous-32 14.3 days
- Sulfur-35 88 days
- Cobalt-57 270 days
- Cobalt-60 5.24 years
- Gold-198 2.7 days
- Mercury-197 2.66 days
- Iron-59 45 days
- Bismuth-214 19.7 minutes
- Plutonium-239 24,000 years
- Lead-214 26.8 minutes
- Mercury-203 46.5 days
- Radon 3.83 days
- Rubidium-86 18.8 days
Equipment: Nuclear Radiation
- Geiger counter
- Simulated brain scan envelopes
- Data for radioactive samples