Inelastic Collisions
Perfectly elastic collisions are those in which no kinetic energy is lost in the collision. Macroscopic collisions are generally inelastic and do not conserve kinetic energy, though of course the total energy is conserved as required by the general principle of conservation of energy. The extreme inelastic collision is one in which the colliding objects stick together after the collision, and this case may be analyzed in general terms: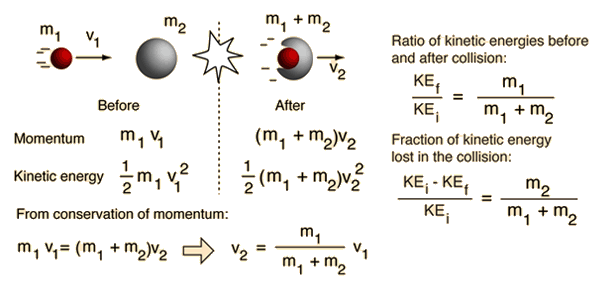
Calculation
Derivation of kinetic energy loss expressions
Examples of inelastic collisions
Collision concepts
| HyperPhysics***** Mechanics | R Nave |
