Fractional Population of Excited States
Fundamental to our understanding of classical molecular phenomena is the Boltzmann distribution, which tells us that the probability that any one molecule will be found with energy E decreases exponentially with energy; i.e., any one molecule is highly unlikely to grab much more than its average share of the total energy available to all the molecules. Mathematically, the Boltzmann distribution can be written in the form
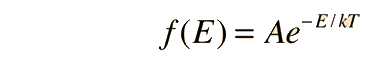 |
|
The fractional population of an excited state can be calculated from the Boltzmann distribution. The distribution of energy depends upon the distribution function and the density of available states for a given energy level. In many cases, there will be more ways to achieve an upper energy level, so that statistical weight must be taken into account in calculating populations.
The example here will not take into account the density of states, but will consider the case of a collection of point particles at thermal equilibrium at temperature T. This can give some insight into the probability of reaching some threshold for a physical phenomenon, or the probability of overcoming some barrier. Note that this is a classical calculation and does not take into account the possibility of quantum mechanical tunneling.
Calculation notes: Default values will be entered for unspecified parameters, but all values can be changed. Default values will be substituted if you put in a fraction greater than one. Otherwise, entering a fraction will cause the temperature to be calculated for the given energy.
| Average thermal energy |
Kinetic theory concepts
| HyperPhysics***** Thermodynamics | R Nave |