Work, Energy and Friction

Example of Energy Balance Calculations

In the "friction experiment" you collected data about distances and times for the masses involved. This data allows you to make calculations of the energies involved in the motions. One of the fundamental symmetries of nature is conservation of energy: "Energy is neither created nor destroyed.". This means that for any physical experiment, you should be able to "balance the energy checkbook" and account for the energies involved in different parts of the experiment. The experiment starts with an energy balance in the form of the gravitational potential energy of the mass hanging on the string. As the mass falls to the floor, you withdraw all of that balance, paying it out in the form of the kinetic energies of the falling mass and the moving block on the table above. The string attached to the block on the wooden runway does work on it which is force x distance. That work moves the block against the frictional resistance mmg where the weight is W=mg . It also gives it kinetic energy so that it is still moving for a time after the hanging mass hits the floor. Ultimately, friction wins out and stops the sliding block, and at that time you can say that all the work done on the block has been "withdrawn" from your energy account as it is dissipated against the frictional resistance. By balancing the energy checkbook, we can imply the work done against friction and determine a value for the coefficient of friction which acted between the block and the wooden track on which it is sliding.

The following is an example analysis from data taken in the laboratory. The detailed analysis has parallel input boxes where you may enter your data for comparison and determine the coefficient of friction from your own data. The data boxes will default to the values for the examples, so you will have to make sure that your values for the measurements are entered.

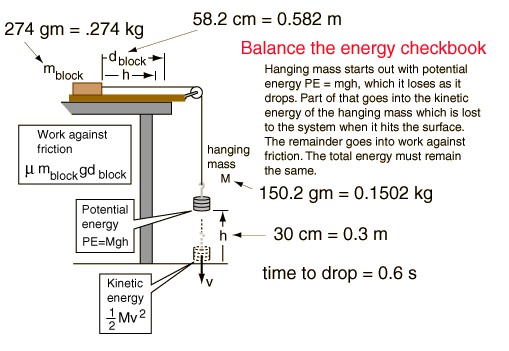
Experimenters: Brett Smith and Julie Griffis

The first step in the analysis is to determine your initial energy balance, the potential energy of the hanging mass.

Potential energy = mgh = (0.1502 kg)(9.8 m/s2)(0.3 m) = 0.44 joules.

Potential energy = mgh = ( kg)(9.8 m/s2)( m) = joules.

The "energy account" that we are considering consists of just the hanging mass and the block to which it is attached by the string. We neglect the string because of its tiny mass, so our account consists of the energy of these two objects. Any energy transferred to the earth (the table, the floor, the wooden track on which it is sliding, etc.) is treated as an energy withdrawal from our system. That energy is of course not lost, since energy is never ultimately lost, but just transferred out of our energy account for the experiment. The first withdrawal occurs when the hanging mass crashes into the floor: it had kinetic energy before the collision, and after the collision it has none. The next step is to calculate that withdrawal.

Since kinetic energy = (1/2)mv2, we need the velocity of impact with the floor. We can calculate the average velocity from the distance divided by the time, but the impact velocity is twice that average. This stage of the energy evaluation is as follows:

vaverage = h/t = 0.30 m/0.6 s = 0.5 m/s

vaverage = h/t = m/ s = m/s

vimpact = 2vaverage = 1 m/s

vimpact = 2vaverage = m/s

Kinetic energy = (1/2)mv2 = (1/2)(0.1502 kg)(1 m/s)2 = 0.075 J

Kinetic energy = (1/2)mv2 = (1/2)( kg)( m/s)2 = J

This amount of energy is now withdrawn from the account, and this completes the energy analysis for the hanging mass. It is sitting on the floor with neither potential or kinetic energy remaining. The remainder of the energy must have been used to do work on the wooden block, the other part of our system. The work done on the wooden block is then

Won block = initial system energy balance - KE lost to floor

Won block = 0.44 J - 0.075 J = 0.365 J

Won block = J - J = J

Since the work done on the block is given by force x distance, and the distance has been measured, the force exerted on the block by the string can be evaluated.

Won block = 0.365 J = Force x distance = F x 0.582 m.

F = 0.365 J/0.582 m = 0.63 Newtons.

F = J/ m = Newtons.

Since the force holding the surfaces together (the normal force) is just the weight of the block, we are now able to evaluate the coefficient of friction.

F = 0.63 N = mmg = m(0.274 kg)(9.8 m/s2) = m2.68 N

The coefficient is then

m = F/mblockg = 0.23

m = ( N) /( kg)g =





Index

Apparatus movie
  HyperPhysics***** NSCI 3001 ***** NSCI 3001 Hands-on exercises Go Back