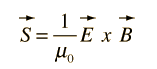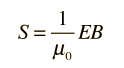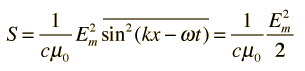Electromagnetic Wave Equation

The wave equation for a plane electric wave traveling in the x direction in space is

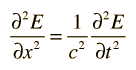
with the same form applying to the magnetic field wave in a plane perpendicular the electric field. Both the electric field and the magnetic field are perpendicular to the direction of travel x. The symbol c represents the speed of light or other electromagnetic waves. The wave equation for electromagnetic waves arises from Maxwell's equations. The form of a plane wave solution for the electric field is

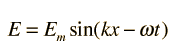
and that for the magnetic field

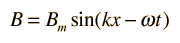
To be consistent with Maxwell's equations, these solutions must be related by

The magnetic field B is perpendicular to the electric field E in the orientation where the vector product E x B is in the direction of the propagation of the wave.

Energy in Electromagnetic Waves

Electromagnetic waves carry energy as they travel through empty space. There is an energy density associated with both the electric field E and the magnetic field B. The rate of energy transport per unit area is described by the vector

which is called the Poynting vector. This expression is a vector product, and since the magnetic field is perpendicular to the electric field, the magnitude can be written

The rate of energy transport S is perpendicular to both E and B and in the direction of propagation of the wave. A condition of the wave solution for a plane wave is B_m = E_m/c so that the average intensity for a plane wave can be written

This makes use of the fact that the average of the square of a sinusoidal function over a whole number of periods is just 1/2.